Three Weinberg College professors named Sloan Research Fellows

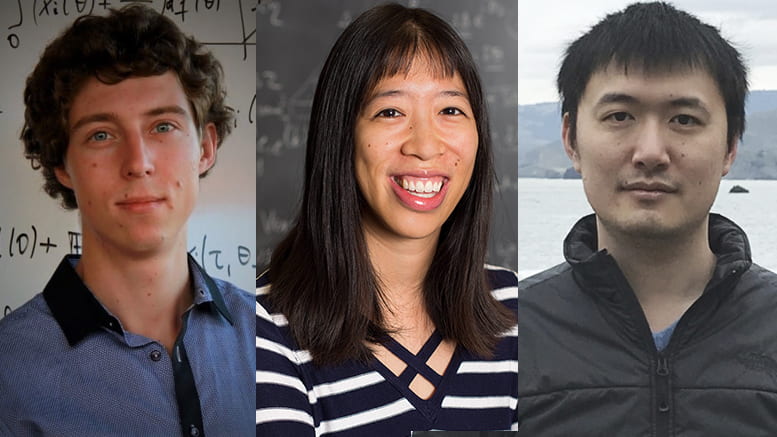
Three Weinberg College faculty members have been awarded a prestigious 2022 Sloan Research Fellowship from the Alfred P. Sloan Foundation. The recipients were economist Piotr Dworczak, astrophysicist Wen-fai Fong, and mathematician Yuchen Liu. They were selected for their creativity, innovation and research accomplishments which make them stand out as the next generation of leaders.

The two-year $75,000 fellowship is one of the most competitive and prestigious awards available to young researchers, and past fellows have gone on to become some of the most decorated experts in their field. The financial support can be used flexibly to advance the fellow’s research.

“Today’s Sloan Research Fellows represent the scientific leaders of tomorrow,” said Adam F. Falk, president of the Alfred P. Sloan Foundation. “As formidable young scholars, they are already shaping the research agenda within their respective fields — and their trailblazing won’t end here.”

Piotr Dworczak was selected as a Sloan Research Fellow in economics. He is an assistant professor of economics in Weinberg College. Dworczak’s research focuses on mechanism and information design, attempting to combine research in pure theory with more applied interests in inequality-aware market design and financial over-the-counter markets.

Selected as a Sloan Research Fellow in physics, Wen-fai Fong is an assistant professor of physics and astronomy in Weinberg and a member of Northwestern’s Center for Interdisciplinary Exploration and Research in Astrophysics(CIERA). Fong’s research group investigates the enigmatic origins of the universe’s fastest explosions, known as transients, and their host galaxy environments. To do this, her team uses observations across the electromagnetic spectrum to study fast radio bursts, gamma-ray bursts, sources of gravitational waves and anything that collides or explodes in outer space.

Yuchen Liu was selected as a Sloan Research Fellow in mathematics. He is an assistant professor of mathematics in Weinberg. Liu’s research primarily focuses on algebraic geometry and its interactions with differential geometry and commutative algebra. His goal is to investigate canonical metrics and moduli spaces of higher dimensional varieties from the viewpoint of algebraic stability.